2015-11-23
10:00-11:00
FAMNIT-POŠTA
Robert Jajcay (UP FAMNIT and Comenius University (Slovakia))
k-regular families of automorphisms and generalized Cayley graphs
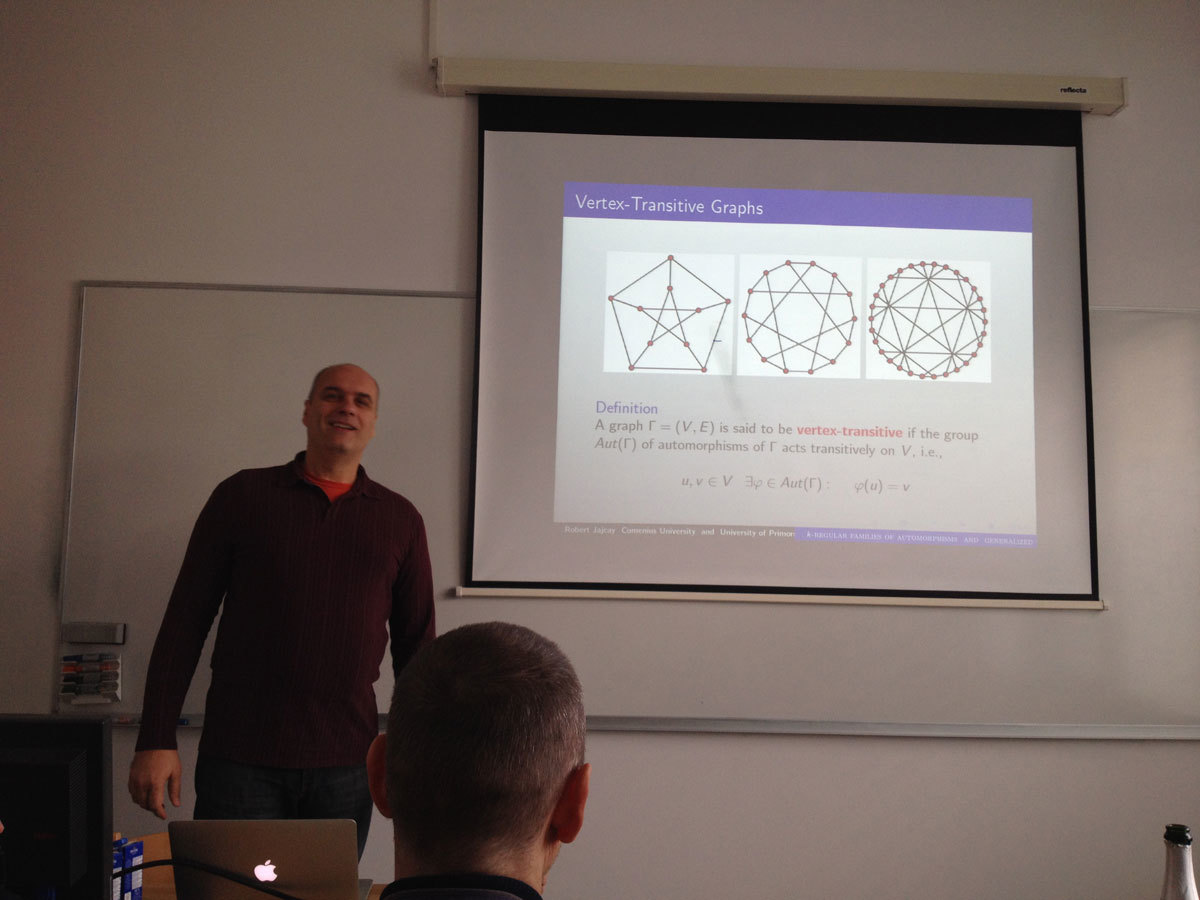
Abstract: A family F of automorphisms of a vertex-transitive graph G is said to be k-regular if for every pair u,v of vertices of G there exist k automorphisms in F mapping u to v. Every vertex-transitive graph admits a k-regular family for some positive k, and quasi-Cayley graphs are vertex-transitive graphs admitting a 1-regular family of automorphisms. We investigate the class of merged Johnson graphs with regard to the parameter k, classify merged Johnson graphs that are Cayley, and relate this topic to that of the existence of a uniform routing.